Understanding Horizontal Asymptotes: A Comprehensive Guide

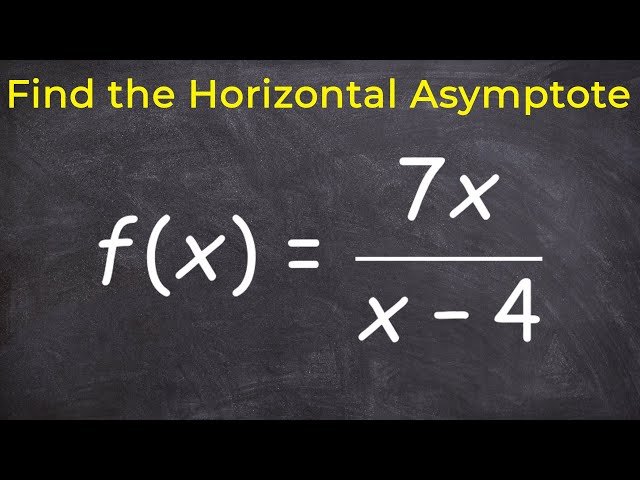
In the realm of calculus and higher mathematics, the concept of asymptotes plays a crucial role in understanding the behavior of functions as they extend towards infinity. Among these, horizontal asymptotes offer insight into the value that a function approaches along the y-axis as the independent variable, typically x, moves towards positive or negative infinity. This guide aims to demystify the process of finding horizontal asymptotes, ensuring that students, educators, and math enthusiasts can easily grasp and apply this concept.

The Essence of Horizontal Asymptotes

Before delving into the methods of finding horizontal asymptotes, it’s essential to understand what they represent. A horizontal asymptote is a horizontal line that the graph of a function approaches but does not necessarily touch or intersect as the value of x approaches infinity (positive or negative). This concept is pivotal in analyzing the end behavior of functions, especially in calculus and mathematical analysis.

Key Principles for Identifying Horizontal Asymptotes

Rational Functions

One of the most common scenarios where horizontal asymptotes are encountered is within rational functions. A rational function is defined as the quotient of two polynomials, expressed as

�(�)=�(�)�(�) f(x)= Q(x) P(x), where �(�) P(x) and �(�) Q(x) are polynomials.

The Degree Matters

The first step in finding horizontal asymptotes in rational functions is to compare the degrees of the numerator and the denominator polynomials. The degree of a polynomial is the highest power of x that appears in the polynomial. Based on this comparison, three primary rules apply:

• If the degree of the numerator is less than the degree of the denominator, the x-axis (y=0) is a horizontal asymptote. This is because as x approaches infinity, the denominator grows faster than the numerator, causing the fraction to reach zero.
• If the degree of the numerator is equal to the degree of the denominator, the function has a horizontal asymptote at
• �=�� y= b a​, where � a and� b are the leading coefficients of the numerator and the denominator, respectively. In this scenario, both the numerator and the denominator grow at the same rate as x approaches infinity, resulting in their ratio approaching the ratio of their leading coefficients.
• If the degree of the numerator is greater than the denominator, there is no horizontal asymptote. This is because the numerator grows without bound in comparison to the denominator, causing the function to approach infinity.

Exponential and Logarithmic Functions

In the case of exponential and logarithmic functions, horizontal asymptotes are determined by the function’s behavior as x approaches positive or negative infinity. For exponential functions, which generally take the form

�=��� y=ab x(where � a and � b are constants, and �>0 b>0), horizontal asymptotes can occur when the function approaches a constant value as x approaches negative infinity, often resulting in �=� y=a as the asymptote if 0<�<1 0<b<1. Logarithmic functions, conversely, typically approach negative infinity as x approaches zero from the positive side, indicating that horizontal asymptotes may not be present in the same way they are for rational or exponential functions.

Non-Rational Functions

Finding horizontal asymptotes may require different approaches for functions that do not fit the mold of rational, exponential, or logarithmic functions. One method is to apply limits at infinity, evaluating

lim⁡�→±∞�(�) Lim X→±∞ f(x) to determine if the function approaches a specific y-value. This approach can be particularly useful for complex functions where direct comparison of polynomial degrees or identifying exponential growth patterns is not applicable.

Practical Applications and Examples

Understanding how to find horizontal asymptotes is not just an academic exercise; it has practical applications in various fields such as physics, engineering, and economics. For instance, in modeling scenarios where growth begins rapidly but eventually levels off, horizontal asymptotes can represent the carrying capacity or maximum sustainable level.

To illustrate, consider the rational function �(�)=2�2+3�−5�2+2�+1f(x)=x2+2x+12x2+3x−5. Applying the rule for when the degrees of the numerator and the denominator are equal, we find that both are of degree 2, and the leading coefficients are 2 and 1, respectively. Therefore, the horizontal asymptote is at �=21=2 y= 12=2.

Conclusion

The pursuit of understanding horizontal asymptotes is a fundamental aspect of calculus that enhances our comprehension of the behavior of functions. By applying the principles outlined in this guide, students and enthusiasts can adeptly navigate the process of identifying horizontal asymptotes across various functions. Whether analyzing the growth trends of a population, the decay rate of a substance, or the behavior of investment returns over time, mastering horizontal asymptotes equips learners with a powerful tool for interpreting and predicting the ultimate behavior of mathematical models.

Frequently Asked Questions about Horizontal Asymptotes

1. What is a horizontal asymptote?

A horizontal asymptote is a horizontal line that a function approaches as the independent variable (usually x) heads towards positive or negative infinity. It describes the behavior of a function at the extremes of the x-axis, indicating the value that the function tends towards but does not necessarily reach or cross.

2. Can a function cross a horizontal asymptote?

Yes, a function can cross a horizontal asymptote. Unlike vertical asymptotes, which are often associated with undefined values of a function, horizontal asymptotes describe the behavior of a function as x approaches infinity. It’s possible for a function to approach a certain value (the horizontal asymptote) and still cross it at some points.

3. How do you find horizontal asymptotes?

The method for finding horizontal asymptotes varies depending on the type of function:

• Rational Functions: Compare the degrees of the numerator and the denominator. If the degree of the numerator is less than the denominator, the horizontal asymptote is y=0. If the degrees are equal, the asymptote is the ratio of the leading coefficients. If the numerator’s degree is greater, there is no horizontal asymptote.
• Exponential and Logarithmic Functions: Evaluate the limit of the function as x approaches positive or negative infinity. The behavior in the limit can indicate the presence of a horizontal asymptote.
• Other Functions: Use limits to determine if the function approaches a specific value as x approaches infinity.